Eccentric Load And Direct Load | Stress Distribution Diagram

Eccentric Load And Direct Load

Eccentric Load

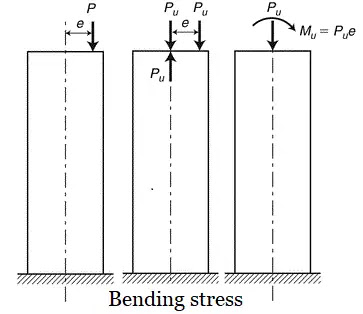
Eccentric load – When the load whose line of action does not directly coincide with the axis of the body then it is called eccentric load. And the distance between the actual axis of the body and the point of loading is called eccentric limit. It always acts on the eccentricity (e) from the centroids of all cross- section of a body.

Eccentricity is denoted by ‘e’.

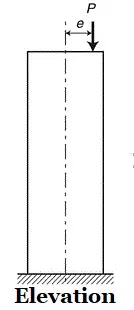
Let us consider a column of a rectangular section having A B C D subjected to an eccentric compressive load P. The load P acts at point E. i.e., on X – X axis. It is eccentric with respect to the Y – Y axis the distance G P is called eccentricity.

Direct Load

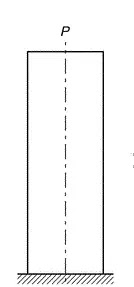
Direct load – When the load whose line of action directly coincides with the axis of the body Then it is called an axial load and direct load. Axial load / direct load is always act on the centroids of the cross – section of a body. [Piles]

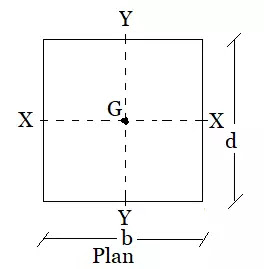
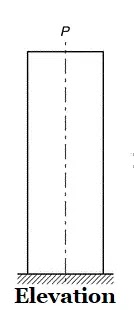
Fig shows the column of a rectangular section of width ‘b’ and thickness ‘d’ subjected to a direct load / axial compressive load. The load ‘P’ acts exactly over the centroid G (Shown in the plan view of a rectangular column). The load P causes a direct stress (σd) of compressive nature whose intensity is uniform throughout the cross – section, Hence the stress distribution diagram at the base of the column is rectangular.

Types Of Direct Load

Direct load is categories between two different loads.

1. Tensile load / Tensile force
2. Compressive load / Compressive force

Tensile Load

Tensile load – When the two equal and opposite forces pull is axially applied on a body, then it is called as tensile load / tensile force the body is subjected to tension which causes increase in the length of a body.

Compressive Load

Compressive load – When the two equal and opposite forces push is axially applied on a body, then it is called as compressive load / compressive force the body is subjected to compression which causes decrease in the length of a body.

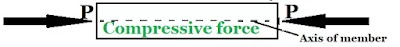
Note – Direct load causes a direct stress, but eccentric load is causes direct as well as bending stress.

---

In eccentric load and direct load term introduced as bending stress and direct stress

---

Bending Stress

Bending Stress – When the load is acting it does not coincide with the axis of the body then, the resistance per unit area is called bending stress.

Bending stress is denoted by ( σb )

     Bending stress ( σb ) =M/I x Y

 Where, M = Calculated bending moment

      I = Moment of inertia around the neutral axis

    Y = Vertical distance away from the neutral axis

Direct Stress

Direct Stress – When the load is acting axially (along the axis) then the resistance per unit area is called direct stress.

         Direct stress ( σd ) = P/A    N/mm sq. 

           Where, P = Compressive load

                       A = Cross–section area (A = B X D)

Stress distribution diagram

1. Bending stress is less then direct stress (σd > σb)
2. Bending stress is equal to the direct stress (σd = σb)
3. Bending stress is more than direct stress (σd < σb)

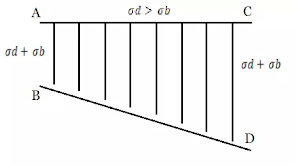
Bending stress is less then direct stress (σd > σb)

If bending stress is less than direct stress (σd > σb) than the stress throughout the section will be of the same nature i.e, compressive nature. The stress distribution diagram is shown below. [Types of piles]

Bending stress is equal to the direct stress (σd = σb)

If bending stress is equal to direct stress (σd = σb) than the stress throughout the section will be of the same nature. i.e, compressive nature. The stress distribution diagram is shown below.+

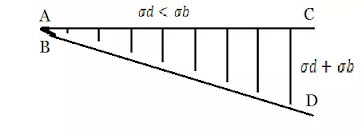
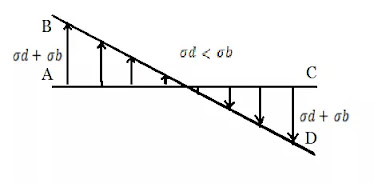
Bending stress is more than direct stress (σd < σb)

If bending stress is more than direct stress (σd < σb) then the stress will be partly tensile and partly compressive both. The stress distribution diagram is shown below.

---